In the Standard Model, the CKM matrix which expresses the probability with which quarks are transformed into different kinds of quark via W boson interactions is described which four parameters which in the Wolfenstein parameterization are called λ, A, ρ¯, and η¯. It is often described in approximated form as follows (in which the "i" is the imaginary number) and the O() term refers to "additional contributions with a combined magnitude on the order of the fourth power of lambda":
The absolute value of the square of each entry is the probability that an up-like quark will transform into a particular down-like quark (and the complex conjugate of the matrix shown above is the probability that a particular down-like quark will transform into a particular up-like quark).
The value of the Wolfenstein parameters and the uncertainties in those measurements as of a 2017 article summarizing the current global averages is as follows:
λ = 0.2251 +0.0004 −0.0004,
A = 0.831 +0.021 −0.031,
ρ¯ = 0.155 +0.008 −0.008,
η¯ = 0.340 +0.010 −0.010.
The global fits discussed in a June 5, 2018 review from the Particle Data Group linked above comes up with slightly different values, the first of which quoted below is based upon frequentists statistics and the second of which is based upon Bayesian statistics (which I would tend to favor in these circumstances).
The global fits discussed in a June 5, 2018 review from the Particle Data Group linked above comes up with slightly different values, the first of which quoted below is based upon frequentists statistics and the second of which is based upon Bayesian statistics (which I would tend to favor in these circumstances).
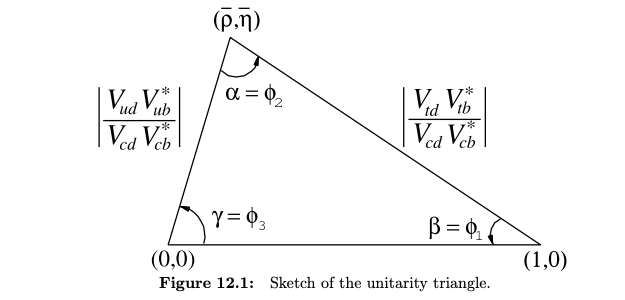
The last of these parameters is the sole source of CP violation in the Standard Model and is one of the more difficult to measure. One way to measure it is to determine the angles that going into the "unitary triangle" shown below (which is over constrained because all three can be determined independently but they must add up to 180º) as it is defined for this purpose, which defines both of the last two parameters of the four Wolfenstein parameterization parameters of the CKM matrix.
via a review article from the Particle Data Group.
A new paper improves the precision with which ρ¯, and η¯ are known.
As the introduction of the paper cited below explains:
Cabibbo-Kobayashi-Maskawa (CKM) matrix, is the central goal of heavy flavor physics program. Specifically, using B decays to determine the three angles α, β and γ of the usual non-squashed unitarity triangle of the CKM matrix respectively and thus to test the closure of the unitarity triangle is a very straightforward and promising way to accomplish this goal. Any discrepancies would suggest possible new sources of CP violation beyond the standard model. In principle, α, β and γ can be determined via measurements of CP violating asymmetry in neutral B decays to CP eigenstates.
The hardest to measure of the three angles in the unitary triangle is gamma. The introduction to the new article cited below continues by explained that:
[I]t is well known that the angle β can be determined in a reliable way with the help of the mixing induced CP violation of a single ”gold-plated” mode B0 → J/ψKS.
Likewise, for α, it can be extracted using neutral B decay, B0 → π +π −, using the isospin symmetry analysis to separate the strong phase difference of tree and penguin contributions by other B → ππ decays.
Theoretically, similar with the measurement of β and α, a straightforward way to obtain of γ might be to use CKM-suppressed B0 s decay, B0 → ρKS, or a analysis for the decays B0 s → D0φ, D¯ 0φ and D0 1φ. However, the observed mixing-induced CP asymmetries are expected to be strongly diluted by the large Bs − B¯ s mixing, so that to determine γ in this way is considerably more involved than β and α.
The third angle γ is currently the least known. It usually depends on strong phase difference of different B decays, which is difficult to calculate reliably. One of the theoretically cleanest way of determining γ is to utilize the interference between the b → cus¯ and b → ucs¯ decay amplitudes with the intermediate states D0 and D¯0 mesons subsequently decay to common final states rather than to use B− → D0KS and B− → D¯0KS decays directly, due to the large uncertainties of the two amplitudes ratio rB and strong phase difference between them.
But, it turns out that using their approach they were able to make great improvements with respect to the status quo in measuring gamma. Before this paper, the latest combination of γ measurements by the LHCb collaboration yielded:
(74.0 +5.0 −5.8 )º.
But, the latest result for CKM angle γ from the paper below is
(69.8 ± 2.1 ± 0.9)º
which implies a combined error of the new result of
(69.8 ± 2.3)º.
The relative error in this hard to measure physical constant is now 3%. Thus, the new paper provide a margin of error that is 57% smaller than the previous margin of error, in addition to dragging down the mean value of the physical constant by about 0.72 sigma (i.e. standard deviations of margin of error in the old value). The new value is consistent with the old world average value at the 0.67 sigma level.
Comparing the margins of error in the previous world average for gamma and the margins of error in the global average value of ρ¯, and η¯, it looks to me like the error margin in these actual CKM parameters will probably be reduced by roughly a third to half of the improvement in the precision of gamma. Thus, the margins of error for ρ¯, and η¯ are likely to decline by about 15%-30% each, with the global fit estimate of ρ¯ going up slightly and the global fit estimate of η¯ going down slightly. Thus, my back of napkin non-rigorous estimate is that the new global averages and margins of errors for the affected Wolfenstein parameters of the CKM matrix after considering this paper might be roughly on the order of:
Comparing the margins of error in the previous world average for gamma and the margins of error in the global average value of ρ¯, and η¯, it looks to me like the error margin in these actual CKM parameters will probably be reduced by roughly a third to half of the improvement in the precision of gamma. Thus, the margins of error for ρ¯, and η¯ are likely to decline by about 15%-30% each, with the global fit estimate of ρ¯ going up slightly and the global fit estimate of η¯ going down slightly. Thus, my back of napkin non-rigorous estimate is that the new global averages and margins of errors for the affected Wolfenstein parameters of the CKM matrix after considering this paper might be roughly on the order of:
ρ¯ = 0.158 +0.006 −0.006,
η¯ = 0.336 +0.008 −0.008.
This isn't a huge improvement in the ultimate bottom line parameter measurements. But, every improvement in the CKM matrix parameters improves the accuracy of every single electroweak calculation going forward, and also improves our ability to distinguish experimentally between background noise in experimental results and beyond the Standard Model signals. If signals are not seen, the exclusions of beyond the Standard Model physics are stronger as a result, and if signals are seen the power of the experiments to see them is greater, all other things being equal.
This isn't a huge improvement in the ultimate bottom line parameter measurements. But, every improvement in the CKM matrix parameters improves the accuracy of every single electroweak calculation going forward, and also improves our ability to distinguish experimentally between background noise in experimental results and beyond the Standard Model signals. If signals are not seen, the exclusions of beyond the Standard Model physics are stronger as a result, and if signals are seen the power of the experiments to see them is greater, all other things being equal.
The paper is:
Extraction of the CKM phaseγ from charmless two-body B meson decays(Submitted on 8 Oct 2019)Utilizing all the experimental measured charmlessB→PP ,
PV
decay modes, where P(V)
denotes a light pseudoscalar (vector) meson, we extract the CKM angle
γ by global fit. All the unknown hadronic parameters are fitted with together from experimental data, so as to make the approach least model dependent. The different contributions for various decay modes are classified by topological weak Feynman diagram amplitudes, which are to be determined by the global fit. To improve the precision of this approach, we consider flavor SU(3) breaking effects of topological diagram amplitudes among different decay modes by including the form factors and decay constants. The fitted result for CKM angle γis $(69.8 \pm 2.1 \pm 0.9) ^{\degree}$. It is consistent with the current world average with a better precision. γ


No comments:
Post a Comment